Example Problem


Problem: H2A is a diprotic acid. Ka1 = 4.7 E-6, Ka2 = 2.7 E-11. A 20.00 mL sample of 0.100 M Na2A solution is titrated with 0.200 M HCl. What will the pH of the solution be after additions of 0, 1, 5, 9.5, 10, 12, 20, and 23 mL of the acid?


Solution: Hey! Wait a second! This isn't fair! Titrating a base with an acid is different from what we're used to! Yep, we know, and Mr. McAfoos knows too, which is why this is fair game for the death test. But before you stab yourself in the heart with your mechanical pencil, take a look at the problem. Everything's the same. Here are the reactions going on in solution:


  1. H2A + H2O --> HA¯ + H3O+
    Ka1
  2. HA¯ + H2O --> A2- + H3O+
    Ka2
  3. A2- + H2O --> HA¯ + OH¯
    Kb1
  4. HA¯ + H2O --> H2A + OH¯
    Kb2
  5. HA¯ + HA¯ --> H2A + A2-
    K


Now let's find the equilibrium constants:


  1. Ka1 = 4.7 E-6
  2. Ka2 = 2.7 E-11
  3. Kb1 = Kw/Ka2 = Kw/2.7 E-11 = 3.7 E-4
  4. Kb2 = Kw/Ka1 = Kw/4.7 E-6 = 2.1 E-9
  5. K = Ka2/Ka1 = 2.7 E-11/4.7 E-6 = 5.7 E-6


Note: Here's the logic behind the equilibrium constant for reaction number 5:


HA¯ + H2O --> A2- + H3O+
Ka2

+
HA¯ + H2O --> H2A + OH¯
Kb2 = Kw/Ka1

+
H3O+ + OH¯ --> 2H2O
1/Kw


_______________________
_________


HA¯ + HA¯ --> H2A + A2-
K


K = (Ka2)(Kw/Ka1)(1/Kw) = Ka2/Ka1


Ba da bing, ba da boom. Now, the equivalence point and the max buffer points:


MBVB = MAVA
(0.100 M)(20.00 mL) = (0.200)(equivalence point)
Equivalence point 1 = 10 mL
Equivalence point 2 = 2 * 10 mL = 20 mL
Max buffer point 1 = (0 + 10)/2 = 5 mL
Max buffer point 2 = (10 + 20)/2 = 15 mL


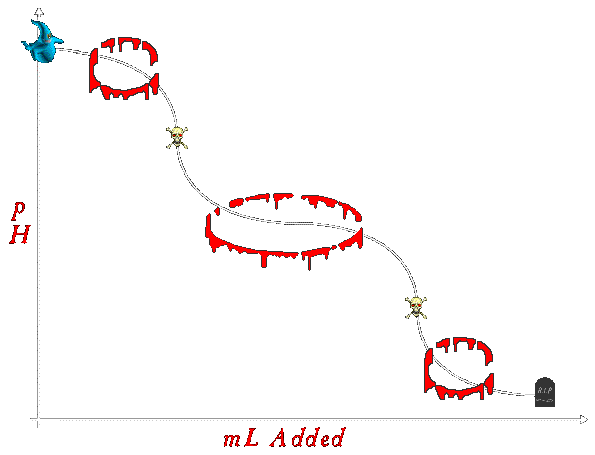
Okay, now that we know all the important points, it's time to draw a spiffy titration curve. I don't know, maybe one like this:

(Click on each section of the graph for the lowdown on what reactions are happening, what the dominant reaction is, and the math for the relevant points)

--Starting Point
--Equivalence Point

--End Point
--Buffer Region
Polyprotic Graph
Start Point

 Stuff in Solution:
A2-, H2O

Reactions:
  1. A2- + H2O --> HA¯ + OH¯
    Kb1 = 3.7 E-4
Dominant Reaction:
A2- + H2O --> HA¯ + OH¯

K of Choice:
3.7 E-4


mL
V (L)
MBVB
MAVA
HA¯
[OH¯]
pH
00.02(0.1)(0.02) =
0.002		0---0 = X2/(0.1 - X) - 3.7 E-4
X = 5.9 E-3		pH=11.8


First Buffer Region

 Stuff in Solution:
A2-, HA¯, H2O

Reactions:
  1. HA¯ + H2O --> A2- + H3O+
    Ka2 = 2.7 E-11
  2. A2- + H2O --> HA¯ + OH¯
    Kb1 = 3.7 E-4
  3. HA¯ + H2O --> H2A + OH¯
    Kb2 = 2.1 E-9
  4. HA¯ + HA¯ --> H2A + A2-
    K = 5.7 E-6
Dominant Reaction:
A2- + H2O --> HA¯ + OH¯

K of Choice:
3.7 E-4


mL
V (L)
MBVB
MAVA
HA¯
pOH
pH
*** Use Henderson/Hasselbach ***
10.0210.002 - 0.0002 =
0.0018		(0.001)(0.2) =
0.0002		0.0002pOH = pKb1 - log(0.0018/0.0002) =
2.5		pH=11.5
*** Max Buffer Point ***
5------------pOH = pKb1 = 3.4pH=10.6
*** We're out of the H/H range--back to equilibrium! ***
9.50.02950.002 - 0.0019 =
0.0001		(0.0095)(0.2) =
0.0019		0.00190 = X(0.0019/0.0295 + X)/(0.0001/0.0295 - X)
[OH¯] = X = 1.9 E-5
pOH = 4.7		pH=9.3


First Equivalence Point

 Here's the deal: for reasons that we still haven't firgured out, and that Mr. McAfoos may never reveal, when you're at an equivalence point between two max buffer points, the pH is equal to the average of the two Ka's, and the pOH is the average of the two Kb's. We don't question it, we just use it. So, at 10 mL:

pOH = (pKb1 + pKb2)/2
pOH = [-log(3.7 E-4) - log(2.1 E-9)]/2
pOH = 6.1
pH = 7.9

Second Buffer Region

 Stuff in Solution:
HA¯, H2A, H2O

Reactions:
  1. H2A + H2O --> HA¯ + H3O+
    Ka1 = 4.7 E-6
  2. HA¯ + H2O --> A2- + H3O+
    Ka2 = 2.7 E-11
  3. HA¯ + H2O --> H2A + OH¯
    Kb2 = 2.1 E-9
  4. HA¯ + HA¯ --> H2A + A2-
    K = 5.7 E-6
Dominant Reaction:
HA¯ + HA¯ --> H2A + A2-

K of Choice:
5.7 E-6


Here's what's happening. After the first equivalence point has been reached, all of the original base has been used up. Now, the unreacted acid reacts with the next level of base until the second equivalence point has been reached.


mL
V (L)
MBVB
MAVA
HA¯
H2A2-
pH
*** Use Henderson/Hasselbach ***
120.0320.002(0.012)(0.2) =
0.0024 - 0.002 =
0.0004		0.002 - 0.0004 =
0.0016		0.0004pH = pKa1 - log(0.0004/0.0016) =
5.9


Second Equivalence Point

 Stuff in Solution:
H2A, H2O

Reactions:
  1. H2A + H2O --> HA¯ + H3O+
    Ka1 = 4.7 E-6
Dominant Reaction:
H2A + H2O --> HA¯ + H3O+

K of Choice:
4.7 E-6


Unfortunately, we can't use the pKa shortcut here, because this equivalence point isn't between two max buffer points. So let's do some equilibrium.


mL
V (L)
MBVB
MAVA
HA¯
H2A2-
pH
200.040.002(0.02)(0.2) =
0.004 - 0.002 =
0.002		0.002 - 0.002 =
0		0.0020 = X2/(0.002/0.04 - X) - 4.7 E-6
[H3O+] = X = 4.8 E-4
pH = 3.3


Third Buffer Region

 There are no titration points in this region, so move on to the endpoint.

End Point

 Stuff in Solution:
H2A, H2O

Reactions:
  1. H2A + H2O --> HA¯ + H3O+
    Ka1 = 4.7 E-6
Dominant Reaction:
H2A + H2O --> HA¯ + H3O+

K of Choice:
4.7 E-6


mL
V (L)
MBVB
MAVA
HA¯
H2A2-
pH
230.0430.002 (0.023)(0.2) =
0.0046 - 0.002 =
0.0026 - 0.002 =
0.0006		0.002 - 0.002 =
0		0.0020 = X(0.0006/0.043 + X)/
(0.002/0.043 - X) - 4.7 E-6




Most excellent! You're through the toughest part of the Death Test. Why don't you go grab Rufus and roll on down to Hydrolysis?


Top Home